The Trigonometry Formula for Double Angles is a continuation of the Sum and Difference of Trigonometry Angles Formula
After we previously studied Formulas for the Sum and Difference of Trigonometry Anglesβ
, we continued the material to Trigonometry Formulas for Double Angles . The double angle in question is 2 Ξ± 2\alpha 2 Ξ± 1 2 Ξ± \dfrac12 \alpha 2 1 β Ξ±
Like the formula for the sum and difference of two angles, the double angle formula is used to determine the trigonometric value for an angle that is not a special angle (0 β , 3 0 β , 4 5 β , 6 0 β , 9 0 β 0^\circ, 30^\circ, 45^\circ, 60^\circ, 90^\circ 0 β , 3 0 β , 4 5 β , 6 0 β , 9 0 β
How to use this double angle formula? see the following review.
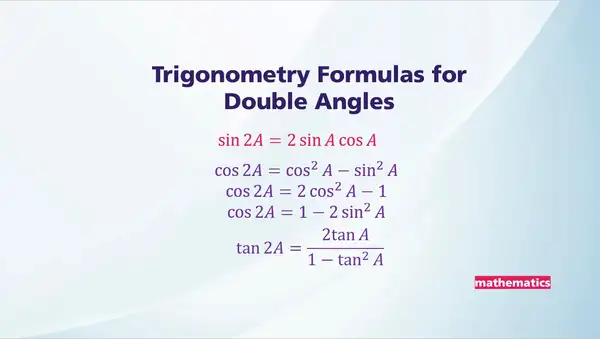
Here are the trigonometric formulas for the double angle sin β‘ 2 Ξ± , cos β‘ 2 Ξ± , tan β‘ 2 Ξ± \sin 2\alpha, \cos 2\alpha, \tan 2\alpha sin 2 Ξ± , cos 2 Ξ± , tan 2 Ξ±
sin β‘ 2 Ξ± = 2 sin β‘ Ξ± cos β‘ Ξ± cos β‘ 2 Ξ± = 2 cos β‘ 2 Ξ± β 1 cos β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± tan β‘ 2 Ξ± = 2 tan β‘ Ξ± 1 β tan β‘ 2 Ξ± \sin 2\alpha=2\sin\alpha \cos\alpha\\ \cos 2\alpha=2\cos^2\alpha-1 \\ \cos 2\alpha = 1-2\sin^2\alpha \\ \tan 2\alpha = \frac{2\tan\alpha}{1-\tan^2\alpha} sin 2 Ξ± = 2 sin Ξ± cos Ξ± cos 2 Ξ± = 2 cos 2 Ξ± β 1 cos 2 Ξ± = 1 β 2 sin 2 Ξ± tan 2 Ξ± = 1 β tan 2 Ξ± 2 tan Ξ± β
We can obtain or prove this formula by using the trigonometry formula for the sum of two angles.
β
\bigstar β
Rumus Sinus sukta rangkap sin β‘ 2 Ξ± = 2 sin β‘ Ξ± cos β‘ Ξ± \sin 2\alpha = 2 \sin \alpha \cos \alpha sin 2 Ξ± = 2 sin Ξ± cos Ξ±
Do not multiply sine roots: sin β‘ ( A + B ) = sin β‘ A cos β‘ B + cos β‘ A sin β‘ B \sin (A+B) = \sin A \cos B + \cos A \sin B sin ( A + B ) = sin A cos B + cos A sin B
sin β‘ 2 Ξ± = sin β‘ ( Ξ± + Ξ± ) = sin β‘ Ξ± cos β‘ Ξ± + cos β‘ Ξ± sin β‘ Ξ± = 2 sin β‘ Ξ± cos β‘ Ξ± \begin{align*}\sin 2\alpha &= \sin ( \alpha + \alpha ) \\ &= \sin \alpha \cos \alpha + \cos \alpha \sin \alpha \\ &= 2 \sin \alpha \cos \alpha \end{align*} sin 2 Ξ± β = sin ( Ξ± + Ξ± ) = sin Ξ± cos Ξ± + cos Ξ± sin Ξ± = 2 sin Ξ± cos Ξ± β
So it is proved: sin β‘ 2 Ξ± = 2 sin β‘ Ξ± cos β‘ Ξ± \sin 2\alpha = 2 \sin \alpha \cos \alpha sin 2 Ξ± = 2 sin Ξ± cos Ξ±
β
\bigstar β
Rumus Cosinus sugat rangkap : cos β‘ 2 Ξ± = cos β‘ 2 Ξ± β sin β‘ 2 Ξ± \cos 2\alpha = \cos ^2 \alpha - \sin ^2 \alpha cos 2 Ξ± = cos 2 Ξ± β sin 2 Ξ±
Ingat rumus cos β‘ ( A + B ) = cos β‘ A cos β‘ B β sin β‘ A sin β‘ B \cos (A + B) = \cos A \cos B - \sin A \sin B cos ( A + B ) = cos A cos B β sin A sin B
cos β‘ 2 Ξ± = cos β‘ ( Ξ± + Ξ± ) = cos β‘ Ξ± cos β‘ Ξ± β sin β‘ Ξ± sin β‘ Ξ± = cos β‘ 2 Ξ± β sin β‘ 2 Ξ± \begin{align*} \cos 2\alpha &= \cos (\alpha + \alpha ) \\ &= \cos \alpha \cos \alpha - \sin \alpha \sin \alpha \\ &= \cos ^2 \alpha - \sin ^2 \alpha \end{align*} cos 2 Ξ± β = cos ( Ξ± + Ξ± ) = cos Ξ± cos Ξ± β sin Ξ± sin Ξ± = cos 2 Ξ± β sin 2 Ξ± β
So it is proven: cos β‘ 2 Ξ± = cos β‘ 2 Ξ± β sin β‘ 2 Ξ± \cos 2\alpha = \cos ^2 \alpha - \sin ^2 \alpha cos 2 Ξ± = cos 2 Ξ± β sin 2 Ξ±
Using the identity formula: sin β‘ 2 A + cos β‘ 2 A = 1 β sin β‘ 2 A = 1 β cos β‘ 2 A \sin ^2 A + \cos ^2 A = 1 \rightarrow \sin ^2 A = 1 - \cos ^2 A sin 2 A + cos 2 A = 1 β sin 2 A = 1 β cos 2 A
β
\bigstar β
Rumus : cos β‘ 2 Ξ± = 2 cos β‘ 2 Ξ± β 1 \cos 2\alpha = 2\cos ^2 \alpha - 1 cos 2 Ξ± = 2 cos 2 Ξ± β 1
cos β‘ 2 Ξ± = cos β‘ 2 Ξ± β sin β‘ 2 Ξ± = cos β‘ 2 Ξ± β ( 1 β cos β‘ 2 Ξ± ) = 2 cos β‘ 2 Ξ± β 1 \begin{align*}\cos 2\alpha &= \cos ^2 \alpha - \sin ^2 \alpha \\ &= \cos ^2 \alpha - (1 - \cos ^2 \alpha ) \\ &= 2\cos ^2 \alpha - 1 \end{align*} cos 2 Ξ± β = cos 2 Ξ± β sin 2 Ξ± = cos 2 Ξ± β ( 1 β cos 2 Ξ± ) = 2 cos 2 Ξ± β 1 β
Terbukti : cos β‘ 2 Ξ± = 2 cos β‘ 2 Ξ± β 1 \cos 2\alpha = 2\cos ^2 \alpha - 1 cos 2 Ξ± = 2 cos 2 Ξ± β 1
β
\bigstar β
Rumus : cos β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± \cos 2\alpha = 1 - 2\sin ^2 \alpha cos 2 Ξ± = 1 β 2 sin 2 Ξ±
cos β‘ 2 Ξ± = cos β‘ 2 Ξ± β sin β‘ 2 Ξ± = ( 1 β sin β‘ 2 Ξ± ) β sin β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± \begin{align*}\cos 2\alpha &= \cos ^2 \alpha - \sin ^2 \alpha \\ &= ( 1 - \sin ^2 \alpha ) - \sin ^2 \alpha \\ &= 1 - 2\sin ^2 \alpha \end{align*} cos 2 Ξ± β = cos 2 Ξ± β sin 2 Ξ± = ( 1 β sin 2 Ξ± ) β sin 2 Ξ± = 1 β 2 sin 2 Ξ± β
Terbukti : cos β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± \cos 2\alpha = 1 - 2\sin ^2 \alpha cos 2 Ξ± = 1 β 2 sin 2 Ξ±
β
\bigstar β
Tangent Double Angle Formula : tan β‘ 2 Ξ± = 2 tan β‘ Ξ± 1 β tan β‘ 2 Ξ± \tan 2\alpha = \frac{2\tan \alpha }{1 - \tan ^2 \alpha } tan 2 Ξ± = 1 β t a n 2 Ξ± 2 t a n Ξ± β
Remember the formula : tan β‘ ( A + B ) = tan β‘ A + tan β‘ B 1 β tan β‘ A tan β‘ B \tan (A + B) = \frac{\tan A + \tan B}{1 - \tan A \tan B} tan ( A + B ) = 1 β t a n A t a n B t a n A + t a n B β
tan β‘ 2 Ξ± = tan β‘ ( Ξ± + Ξ± ) = tan β‘ Ξ± + tan β‘ Ξ± 1 β tan β‘ Ξ± tan β‘ Ξ± = 2 tan β‘ Ξ± 1 β tan β‘ 2 Ξ± \begin{align*} \tan 2\alpha &= \tan ( \alpha + \alpha ) \\ &= \frac{\tan \alpha + \tan \alpha }{1 - \tan \alpha \tan \alpha } \\ &= \frac{2\tan \alpha }{1 - \tan ^2 \alpha } \end{align*} tan 2 Ξ± β = tan ( Ξ± + Ξ± ) = 1 β tan Ξ± tan Ξ± tan Ξ± + tan Ξ± β = 1 β tan 2 Ξ± 2 tan Ξ± β β
So it is proved: tan β‘ 2 Ξ± = 2 tan β‘ Ξ± 1 β tan β‘ 2 Ξ± \tan 2\alpha = \dfrac{2\tan \alpha }{1 - \tan ^2 \alpha } tan 2 Ξ± = 1 β tan 2 Ξ± 2 tan Ξ± β
Examples of Compound Angle Questions Simplify the following shapes
2 sin β‘ 22. 5 β cos β‘ 22. 5 β 2 \sin 22.5^\circ \cos 22.5^\circ 2 sin 22. 5 β cos 22. 5 β 2 cos β‘ 2 67 , 5 β β 1 2 \cos^2 67,5^\circ β 1 2 cos 2 67 , 5 β β1 2 tan β‘ 3 Ξ± 1 β tan β‘ 2 Ξ± \dfrac{2\tan 3\alpha}{1-\tan^2\alpha} 1 β tan 2 Ξ± 2 tan 3 Ξ± β Solution
2 sin β‘ 22. 5 β cos β‘ 22. 5 β 2 \sin 22.5^\circ \cos 22.5^\circ 2 sin 22. 5 β cos 22. 5 β sin β‘ 2 A = 2 sin β‘ A cos β‘ A \sin 2A=2\sin A \cos A sin 2 A = 2 sin A cos A 2 sin β‘ 22 , 5 β cos β‘ 22 , 5 β = sin β‘ 2 ( 22 , 5 β ) = sin β‘ 4 5 β = 1 2 2 \begin{align*}2 \sin 22,5^\circ \cos 22,5^\circ&=\sin 2(22,5^\circ)\\&=\sin 45^\circ \\ &=\frac12 \sqrt{2}\end{align*} 2 sin 22 , 5 β cos 22 , 5 β β = sin 2 ( 22 , 5 β ) = sin 4 5 β = 2 1 β 2 β β 2 cos β‘ 2 67 , 5 β β 1 2 \cos^2 67,5^\circ β 1 2 cos 2 67 , 5 β β1 cos β‘ 2 A = 2 cos β‘ 2 A β 1 \cos 2A=2\cos^2 A -1 cos 2 A = 2 cos 2 A β 1 2 cos β‘ 2 67 , 5 β β 1 = cos β‘ 2 ( 67 , 5 β ) = cos β‘ 13 5 β = cos β‘ ( 18 0 β β 4 5 β ) = β cos β‘ 4 5 β = β 1 2 2 \begin{align*}2 \cos^2 67,5^\circ β 1&=\cos 2(67,5^\circ)\\&=\cos 135^\circ = \cos (180^\circ - 45^\circ) \\ &=-\cos 45^\circ =-\frac12 \sqrt{2}\end{align*} 2 cos 2 67 , 5 β β1 β = cos 2 ( 67 , 5 β ) = cos 13 5 β = cos ( 18 0 β β 4 5 β ) = β cos 4 5 β = β 2 1 β 2 β β 2 tan β‘ 3 Ξ± 1 β tan β‘ 2 Ξ± \dfrac{2\tan 3\alpha}{1-\tan^2\alpha} 1 β tan 2 Ξ± 2 tan 3 Ξ± β tan β‘ 2 A = 2 tan β‘ A 1 β tan β‘ 2 A \tan 2A=\dfrac{2\tan A}{1-\tan^2A} tan 2 A = 1 β tan 2 A 2 tan A β 2 tan β‘ 3 Ξ± 1 β tan β‘ 2 Ξ± = tan β‘ 2 ( 3 Ξ± ) = tan β‘ 6 Ξ± \begin{align*}\frac{2\tan 3\alpha}{1- \tan^2 \alpha }&=\tan 2(3\alpha) \\ &=\tan 6\alpha \end{align*} 1 β tan 2 Ξ± 2 tan 3 Ξ± β β = tan 2 ( 3 Ξ± ) = tan 6 Ξ± β Given sin β‘ A = 5 13 \sin A =\dfrac{5}{13} sin A = 13 5 β sin β‘ 2 A \sin 2A sin 2 A cos β‘ 2 A \cos 2A cos 2 A tan β‘ 2 A \tan 2A tan 2 A
Solution
Because sin β‘ A \sin A sin A cos β‘ A \cos A cos A
We just use the identity formula, namely cos β‘ A = 1 β sin β‘ 2 A \cos A = \sqrt{1-\sin^2A} cos A = 1 β sin 2 A β
sin β‘ A = 5 13 \sin A =\dfrac{5}{13} sin A = 13 5 β
cos β‘ A = 1 β sin β‘ 2 A = 1 β ( 5 13 ) 2 = 1 β 25 169 = 144 169 cos β‘ A = 12 13 \begin{align*} \cos A &= \sqrt{1-\sin^2A}\\ &= \sqrt{1-\left( \frac{5}{13} \right)^2} \\ &=\sqrt{1- \frac{25}{169} } \\ &=\sqrt{\frac{144}{169}} \\ \cos A &= \frac{12}{13}\end{align*} cos A cos A β = 1 β sin 2 A β = 1 β ( 13 5 β ) 2 β = 1 β 169 25 β β = 169 144 β β = 13 12 β β
Nilai sin β‘ 2 A \sin 2A sin 2 A
sin β‘ 2 A = 2 sin β‘ A cos β‘ A = 2 β
5 13 β
12 13 sin β‘ 2 A = 120 169 \begin{align*}\sin 2 A &=2\sin A \cos A \\ &= 2 \cdot \frac{5}{13} \cdot \frac{12}{13} \\ \sin 2A&= \frac{120}{169}\end{align*} sin 2 A sin 2 A β = 2 sin A cos A = 2 β
13 5 β β
13 12 β = 169 120 β β
Nilai cos β‘ 2 A \cos 2A cos 2 A
cos β‘ 2 A = cos β‘ 2 A β sin β‘ 2 A = ( 12 13 ) 2 β ( 5 13 ) 2 = 144 169 β 25 169 cos β‘ 2 A = 119 169 \begin{align*}\cos 2 A &=\cos^2 A - \sin^2 A \\ &= \left(\frac{12}{13} \right)^2- \left(\frac{5}{13} \right)^2\\ &= \frac{144}{169}-\frac{25}{169}\\ \cos 2A &= \frac{119}{169} \end{align*} cos 2 A cos 2 A β = cos 2 A β sin 2 A = ( 13 12 β ) 2 β ( 13 5 β ) 2 = 169 144 β β 169 25 β = 169 119 β β
Nilai tan β‘ 2 A \tan 2A tan 2 A
tan β‘ 2 A = sin β‘ 2 A cos β‘ 2 A = 120 169 119 169 tan β‘ 2 A = 120 119 \begin{align*}\tan 2A &=\frac{\sin 2A}{\cos 2A} \\ &=\frac{\frac{120}{169}}{\frac{119}{169}} \\ \tan 2A &=\frac{120}{119} \end{align*} tan 2 A tan 2 A β = cos 2 A sin 2 A β = 169 119 β 169 120 β β = 119 120 β β
From the double angle trigonometry formula, the trigonometry formula for
half an angle, namely by setting 1 2 Ξ± \dfrac12 \alpha 2 1 β Ξ± Ξ± \alpha Ξ±
Trigonometry Formulas for sin β‘ 1 2 A , cos β‘ 1 2 A , \sin \frac{1}{2} A , \cos \frac{1}{2} A, sin 2 1 β A , cos 2 1 β A , tan β‘ 1 2 A \tan \frac{1}{2} A tan 2 1 β A
sin β‘ 1 2 A = 1 β cos β‘ A 2 cos β‘ 1 2 A = 1 + cos β‘ A 2 tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A = sin β‘ A 1 + cos β‘ A = 1 β cos β‘ A sin β‘ A \begin{align*} \sin \frac{1}{2} A & = \sqrt{\frac{1- \cos A}{2}} \\ \cos \frac{1}{2} A & = \sqrt{\frac{1 + \cos A}{2}} \\ \tan \frac{1}{2} A & = \sqrt{\frac{1 - \cos A}{1 + \cos A}} = \frac{\sin A}{1+ \cos A} = \frac{1- \cos A}{\sin A } \end{align*} sin 2 1 β A cos 2 1 β A tan 2 1 β A β = 2 1 β cos A β β = 2 1 + cos A β β = 1 + cos A 1 β cos A β β = 1 + cos A sin A β = sin A 1 β cos A β β
Misalkan 2 Ξ± = A β Ξ± = 1 2 A 2\alpha = A \rightarrow \alpha = \frac{1}{2} A 2 Ξ± = A β Ξ± = 2 1 β A
Substitute the form of the example above into the double angle trigonometric equation that will be proven.
β
\bigstar β
Rumus : sin β‘ 1 2 A = 1 β cos β‘ A 2 \sin \frac{1}{2} A = \sqrt{\frac{1- \cos A}{2}} sin 2 1 β A = 2 1 β c o s A β β
use the formula: cos β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± \cos 2 \alpha = 1 - 2\sin ^2 \alpha cos 2 Ξ± = 1 β 2 sin 2 Ξ±
cos β‘ 2 Ξ± = 1 β 2 sin β‘ 2 Ξ± cos β‘ A = 1 β 2 sin β‘ 2 1 2 A 2 sin β‘ 2 1 2 A = 1 β cos β‘ A sin β‘ 2 1 2 A = 1 β cos β‘ A 2 sin β‘ 1 2 A = 1 β cos β‘ A 2 \begin{align*}\cos 2 \alpha &= 1 - 2\sin ^2 \alpha \\ \cos A &= 1 - 2\sin ^2 \frac{1}{2} A\\2\sin ^2 \frac{1}{2} A &= 1 - \cos A \\\sin ^2 \frac{1}{2} A &= \frac{1 - \cos A}{2} \\\sin \frac{1}{2} A &= \sqrt{\frac{1 - \cos A}{2} } \end{align*} cos 2 Ξ± cos A 2 sin 2 2 1 β A sin 2 2 1 β A sin 2 1 β A β = 1 β 2 sin 2 Ξ± = 1 β 2 sin 2 2 1 β A = 1 β cos A = 2 1 β cos A β = 2 1 β cos A β β β
Until proven : sin β‘ 1 2 A = 1 β cos β‘ A 2 \sin \frac{1}{2} A = \sqrt{\frac{1- \cos A}{2}} sin 2 1 β A = 2 1 β c o s A β β
β
\bigstar β
Rumus : cos β‘ 1 2 A = 1 + cos β‘ A 2 \cos \frac{1}{2} A = \sqrt{\frac{1 + \cos A}{2}} cos 2 1 β A = 2 1 + c o s A β β
use the formula: cos β‘ 2 Ξ± = 2 cos β‘ 2 Ξ± β 1 \cos 2 \alpha = 2\cos ^2 \alpha - 1 cos 2 Ξ± = 2 cos 2 Ξ± β 1
cos β‘ 2 Ξ± = 2 cos β‘ 2 Ξ± β 1 cos β‘ A = 2 cos β‘ 2 1 2 A β 1 2 cos β‘ 2 1 2 A = 1 + cos β‘ A cos β‘ 2 1 2 A = 1 + cos β‘ A 2 cos β‘ 1 2 A = 1 + cos β‘ A 2 \begin{align*} \cos 2 \alpha &= 2\cos ^2 \alpha - 1 \\\cos A &= 2\cos ^2 \frac{1}{2}A - 1 \\2\cos ^2 \frac{1}{2}A &= 1 + \cos A \\\cos ^2 \frac{1}{2}A &= \frac{1 + \cos A}{2} \\\cos \frac{1}{2}A &= \sqrt{\frac{1 + \cos A}{2} } \end{align*} cos 2 Ξ± cos A 2 cos 2 2 1 β A cos 2 2 1 β A cos 2 1 β A β = 2 cos 2 Ξ± β 1 = 2 cos 2 2 1 β A β 1 = 1 + cos A = 2 1 + cos A β = 2 1 + cos A β β β
Until proven : cos β‘ 1 2 A = 1 + cos β‘ A 2 \cos \frac{1}{2} A = \sqrt{\frac{1 + \cos A}{2}} cos 2 1 β A = 2 1 + c o s A β β
β
\bigstar β
Rumus : tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A = sin β‘ A 1 + cos β‘ A = 1 β cos β‘ A sin β‘ A \tan \frac{1}{2} A = \sqrt{\frac{1 - \cos A}{1 + \cos A}} = \frac{\sin A }{1+ \cos A} = \frac{1- \cos A}{\sin A } tan 2 1 β A = 1 + c o s A 1 β c o s A β β = 1 + c o s A s i n A β = s i n A 1 β c o s A β
gunakan rumus : tan β‘ 1 2 A = sin β‘ 1 2 A cos β‘ 1 2 A , sin β‘ 1 2 A = 1 β cos β‘ A 2 \tan \frac{1}{2}A = \frac{\sin \frac{1}{2}A }{\cos \frac{1}{2}A } , \sin \frac{1}{2} A = \sqrt{\frac{1- \cos A}{2}} tan 2 1 β A = c o s 2 1 β A s i n 2 1 β A β , sin 2 1 β A = 2 1 β c o s A β β cos β‘ 1 2 A = 1 + cos β‘ A 2 \cos \frac{1}{2} A = \sqrt{\frac{1 + \cos A}{2}} cos 2 1 β A = 2 1 + c o s A β β
to). Rumus Pertama: tan β‘ 1 2 A = sin β‘ 1 2 A cos β‘ 1 2 A \tan \frac{1}{2}A = \frac{\sin \frac{1}{2}A }{\cos \frac{1}{2}A } tan 2 1 β A = c o s 2 1 β A s i n 2 1 β A β
tan β‘ 1 2 A = sin β‘ 1 2 A cos β‘ 1 2 A = 1 β cos β‘ A 2 1 + cos β‘ A 2 tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A \begin{align*}\tan \frac{1}{2} A &= \frac{\sin \frac{1}{2}A }{\cos \frac{1}{2}A } \ &= \frac{ \sqrt{\frac{1- \cos A}{2}} }{ \sqrt{\frac{1 + \cos A}{2}} } \ \tan \frac{1}{2}A &= \sqrt{ \frac{1- \cos A}{1 + \cos A} } \end{align*} tan 2 1 β A β = cos 2 1 β A sin 2 1 β A β β = 2 1 + c o s A β β 2 1 β c o s A β β β tan 2 1 β A β = 1 + cos A 1 β cos A β β β
b). Second formula:
tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A Γ 1 + cos β‘ A 1 + cos β‘ A = 1 β cos β‘ 2 A ( 1 + cos β‘ A ) 2 = sin β‘ 2 A ( 1 + cos β‘ A ) 2 = sin β‘ A 1 + cos β‘ A \begin{align*} \tan \frac{1}{2}A &= \sqrt{ \frac{1- \cos A}{1 + \cos A} } \\ \tan \frac{1}{2}A &= \sqrt{ \frac{1- \cos A}{1 + \cos A} \times \frac{1 + \cos A}{1 + \cos A} } \\ &= \sqrt{ \frac{1- \cos ^2 A}{(1 + \cos A)^2} } \\ &= \sqrt{ \frac{\sin ^2 A }{(1 + \cos A)^2} } \\ &= \frac{\sin A}{1+ \cos A} \end{align*} tan 2 1 β A tan 2 1 β A β = 1 + cos A 1 β cos A β β = 1 + cos A 1 β cos A β Γ 1 + cos A 1 + cos A β β = ( 1 + cos A ) 2 1 β cos 2 A β β = ( 1 + cos A ) 2 sin 2 A β β = 1 + cos A sin A β β
c). Third formula:
tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A Γ 1 β cos β‘ A 1 β cos β‘ A = ( 1 β cos β‘ A ) 2 1 β cos β‘ 2 A = ( 1 β cos β‘ A ) 2 sin β‘ 2 A = 1 β cos β‘ A sin β‘ A \begin{align*} \tan \frac{1}{2}A &= \sqrt{\frac{1- \cos A}{1 + \cos A} } \\ \tan \frac{1}{2}A &= \sqrt{\frac{1- \cos A}{1 + \cos A} \times \frac{1 - \cos A}{1 - \cos A} } \\ &= \sqrt{\frac{(1- \cos A)^2}{1 - \cos ^2 A} } \\ &= \sqrt{\frac{(1- \cos A)^2}{\sin ^2 A } } \\ &= \frac{1- \cos A}{\sin A } \end{align*} tan 2 1 β A tan 2 1 β A β = 1 + cos A 1 β cos A β β = 1 + cos A 1 β cos A β Γ 1 β cos A 1 β cos A β β = 1 β cos 2 A ( 1 β cos A ) 2 β β = sin 2 A ( 1 β cos A ) 2 β β = sin A 1 β cos A β β
Sehingga terbukti: tan β‘ 1 2 A = 1 β cos β‘ A 1 + cos β‘ A = sin β‘ A 1 + cos β‘ A = 1 β cos β‘ A sin β‘ A \tan \frac{1}{2} A = \sqrt{\frac{1 - \cos A}{1 + \cos A}} = \frac{\sin A}{1+ \cos A } = \frac{1- \cos A}{\sin A } tan 2 1 β A = 1 + c o s A 1 β c o s A β β = 1 + c o s A s i n A β = s i n A 1 β c o s A β
Example of a Half-Angle Question: Calculate the value of:
sin β‘ 1 5 β \sin 15^\circ sin 1 5 β cos β‘ 67 , 5 β \cos 67,5^\circ cos 67 , 5 β tan β‘ 22. 5 β \tan 22.5^\circ tan 22. 5 β Alternative Solution:
The value of sin β‘ 1 5 β \sin 15^\circ sin 1 5 β
Let 1 2 A = 1 5 β β A = 3 0 β \frac{1}{2}A = 15^\circ \rightarrow A = 30^\circ 2 1 β A = 1 5 β β A = 3 0 β
sin β‘ 1 2 A = 1 β cos β‘ A 2 sin β‘ 1 5 β = 1 β cos β‘ 3 0 β 2 = 1 β 1 2 3 2 = 2 β 3 4 = 1 2 2 β 3 \begin{align*}\sin \frac{1}{2} A &= \sqrt{\frac{1 - \cos A}{2} } \\ \sin 15^\circ &= \sqrt{\frac{1 - \cos 30^\circ}{2} } \\ &= \sqrt{\frac{1 - \frac{1}{2} \sqrt{3} }{2} } \\ &= \sqrt{\frac{2 - \sqrt{3} }{4} } \\ &= \frac{1}{2} \sqrt{2 - \sqrt{3} } \end{align*} sin 2 1 β A sin 1 5 β β = 2 1 β cos A β β = 2 1 β cos 3 0 β β β = 2 1 β 2 1 β 3 β β β = 4 2 β 3 β β β = 2 1 β 2 β 3 β β β
So, the value sin β‘ 1 5 β = 1 2 2 β 3 \sin 15^\circ = \frac{1}{2} \sqrt{2 - \sqrt{3} } sin 1 5 β = 2 1 β 2 β 3 β β
The value of cos β‘ 67. 5 β \cos 67.5^\circ cos 67. 5 β
Let 1 2 A = 67. 5 β β A = 13 5 β \frac{1}{2}A = 67.5^\circ \rightarrow A = 135^\circ 2 1 β A = 67. 5 β β A = 13 5 β
nilai cos β‘ 13 5 β = cos β‘ ( 18 0 β β 4 5 β ) = β cos β‘ 4 5 β = β 1 2 2 \cos 135^\circ = \cos ( 180^\circ - 45^\circ ) = -\cos 45^\circ = -\frac{1}{2} \sqrt{2} cos 13 5 β = cos ( 18 0 β β 4 5 β ) = β cos 4 5 β = β 2 1 β 2 β
cos β‘ 1 2 A = 1 + cos β‘ A 2 cos β‘ 67 , 5 β = 1 + cos β‘ 13 5 β 2 = 1 + ( β 1 2 2 ) 2 = 2 β 2 4 = 1 2 2 β 2 \begin{align*}\cos \frac{1}{2} A &= \sqrt{\frac{1 + \cos A}{2} } \\ \cos 67,5^\circ &= \sqrt{\frac{1 + \cos 135^\circ}{2} } \\ &= \sqrt{\frac{1 + (-\frac{1}{2} \sqrt{2} )}{2} } \\ &= \sqrt{\frac{2 - \sqrt{2} }{4} } \\ &= \frac{1}{2} \sqrt{2 - \sqrt{2} } \end{align*} cos 2 1 β A cos 67 , 5 β β = 2 1 + cos A β β = 2 1 + cos 13 5 β β β = 2 1 + ( β 2 1 β 2 β ) β β = 4 2 β 2 β β β = 2 1 β 2 β 2 β β β
So, the value of cos β‘ 67. 5 β = 1 2 2 β 2 \cos 67.5^\circ = \frac{1}{2} \sqrt{2 - \sqrt{2} } cos 67. 5 β = 2 1 β 2 β 2 β β
The value of tan β‘ 22. 5 β \tan 22.5^\circ tan 22. 5 β
Let 1 2 A = 22. 5 β β A = 4 5 β \frac{1}{2}A = 22.5^\circ \rightarrow A = 45^\circ 2 1 β A = 22. 5 β β A = 4 5 β
tan β‘ 1 2 A = sin β‘ A 1 + cos β‘ A tan β‘ 22 , 5 β = sin β‘ 4 5 β 1 + cos β‘ 4 5 β = 1 2 2 1 + 1 2 2 = 2 2 + 2 = 2 2 + 2 Γ 2 β 2 2 β 2 = 2 2 β 2 4 β 2 = 2 2 β 2 2 = 2 β 1 \begin{align*} \tan \frac{1}{2} A &= \frac{\sin A}{1+ \cos A} \\ \tan 22,5^\circ &= \frac{\sin 45^\circ}{1+ \cos 45^\circ} \\ &= \frac{\frac{1}{2} \sqrt{2} }{1+ \frac{1}{2} \sqrt{2} } \\ &= \frac{ \sqrt{2} }{2+ \sqrt{2} } \\ &= \frac{ \sqrt{2} }{2+ \sqrt{2} } \times \frac{2 - \sqrt{2} }{2 - \sqrt{2} } \\ &= \frac{ 2\sqrt{2} - 2 }{4 - 2} \\ &= \frac{ 2\sqrt{2} - 2 }{2} \\ &= \sqrt{2} - 1 \end{align*} tan 2 1 β A tan 22 , 5 β β = 1 + cos A sin A β = 1 + cos 4 5 β sin 4 5 β β = 1 + 2 1 β 2 β 2 1 β 2 β β = 2 + 2 β 2 β β = 2 + 2 β 2 β β Γ 2 β 2 β 2 β 2 β β = 4 β 2 2 2 β β 2 β = 2 2 2 β β 2 β = 2 β β 1 β
Thus, the value tan β‘ 22. 5 β = 2 β 1 \tan 22.5^\circ = \sqrt{2} - 1 tan 22. 5 β = 2 β β 1
